You want to minimize beam deflection. If you had to choose between changing the width or the height of the cross-section of a beam, which would one you choose and why?

hardwarefyi · July 29, 2020, 6:11am

Deflection = \frac{PL}{EI}

hardwarefyi · August 4, 2020, 11:16pm

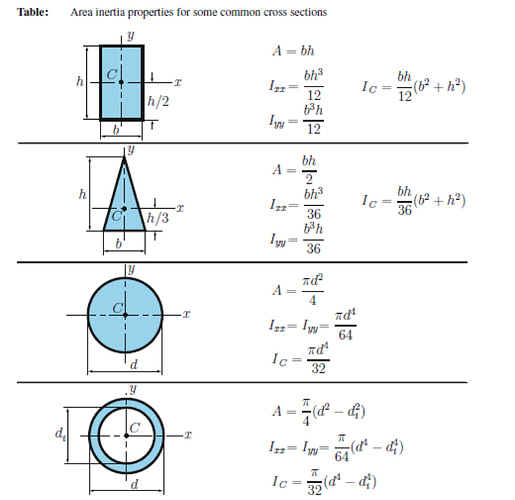
In any deflection equation, the cross-section of a beam is related to the area moment of inertia term. A key concept. Take the example of a rectangular cross-section, with the area moment of inertia being

I_{xx} = bh^3/12

Simply by looking at the equation, we know that changing the height would have a greater influence due to the quantity being tripled. This equation is applicable for the orientation of the rectangle shown in the image below.

If the rectangle was flipped on its side so that the h length is facing down,

I_{yy} = b^3h/12

would be applicable.